The ratio is defined as the quantitative relation between two values showing the number of times one value contains or is contained within the other.
The ratio in the mathematical term used to compare two similar quantities expressed in the same units.
The ratio of two numbers ‘x’ and ‘y’ is denoted as x:y
Note: Fractions and ratio are same but the only difference is that ratio is unit less quantity but fraction is not.
We cannot use x for solving

Total parts = 4 + 5 = 9
First number = `(\frac { 4 }{ 9 })`900 = 400
Second number = `(\frac { 5 }{ 9 })`900 = 500
2. Divide 1500 in the ratio 5:7:3
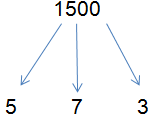
Total parts = 5 + 7 + 3 = 15
First number = `(\frac { 5 }{ 15 })`1500 = 500
Second number = `(\frac { 7 }{ 15 })`1500 = 700
Third number = `(\frac { 3 }{ 15 })`1500 = 300
Solution:
As two parameters which are weight and cost are directly proportional.
Hence, `\frac { W1 }{ W2 } = \frac { C1 }{ C2 } `
`\frac { 3 }{ 8 } = \frac { 45000 }{ C2 } `3/8 = 45000/C2
C2 = 120000
If parameter A and B are inversely proportional then they will satisfy the following equation
`\frac { A1 }{ A2 } = \frac { B1 }{ B2 } `
\begin{matrix} A: & B: & C \\ x & y & y \\ m & m & n \end{matrix}
A: B: C → x` \times ` m : y ` \times ` m : y ` \times ` n
\begin{matrix} A: & B: & C \\ 4 & 5 & 5 \\ 3 & 3 & 4 \end{matrix}
A:B:C → 4` \times `3 : 5` \times `3 : 5` \times `4
A:B:C → 12:15:20
If the ratio of a salary of A and B is 2:3, B and C is 4:5, C and D is 6:7. If the salary of A is 48000 then Find the salary of D.
\begin{matrix} A: & B: & C: & D \\ 2 & 3 & 3 & 3 \\ 4 & 4 & 5 & 5 \\ 6 & 6 & 6 & 7\end{matrix}
A:B:C:D à 48: 72: 90: 105
A:D = 48:105
`\frac { A }{ D }` = `\frac { 48 }{ 105 }`
`\frac { 48000 }{ D }` = `\frac { 48 }{ 105 }`
D’s salary = 105000
Ratio ⟶ 3: 5
Assume the actual number 3x and 5x
According to the question,
`(3x)^{ 2 }` + `(5x)^{ 2 }` = 30600
`34x^{ 2 }` = 30600
`x^{ 2 }` = 900
x = 30
Substitute the value of x in 3x and 5x to find numbers
Hence the numbers are 90 and 150
Value Ratio⟶ V1 : V2 : V3
(Q1` \times `V1) + (Q2` \times `V2) + (Q3` \times `V3) = T
Common factor X = Total Amount/ T
Quantity of each coin = Q1X , Q2X, Q3X
Solutions:
T = (5` \times `2) + (10` \times `4) + (20` \times `5)
T = 150 paisa = Rs. 1.50
X = `\frac { 4.50 }{ 1.50 }`4.50/ 1.50 = 3
Hence the quantity of 20 paisa coins = 5 ` \times ` 3 = 15 coins
2. The bag contains 440 coins of 1 rupee, 50 paisa and 25 paisa and their value are in the ratio 6:7:9. Find the number of 50 paisa coins.
Solution:
Value Ratio ⟶ 6:7:9
Coins Ratio ⟶ 6` \times `1: 7` \times `2: 9` \times `4
3:7:18
Number of 50 paisa coins = `(\frac { 7 }{ 28 }) \times ` 440 = 110
Solution:
Let us consider the length of bigger part be 1 m and the smaller part be ‘x’ m.
So length of full stick = (1+x) m
Acc. to the ques.
`\frac { 1 }{ x } ` = `\frac { 1 + x }{ x } `
`x^{ 2 }` + x = 1
x = `\frac { (-1+\sqrt { 5 } ) }{ 2 }` (As the length will be positive)
Solution:
Let us suppose the salary of A be Rs. x, B be Rs. y and C be Rs. Z
Then the savings of them is
`\frac { (x\times 25)}{100}`, `\frac { (y\times 15)}{100}`, `\frac { (Z\times 20)}{100}`
`\frac { x }{ 4 }` , `\frac { 3y }{ 20 }`, `\frac { z }{ 5 }` = 8:9:20
x:y:z = 32:60:100 = 8:15:25
B’s salary will be `(\frac { 15 }{ 48 })\times ` 96000 = Rs. 30000
Solution:
Initial ratio of seats = 5:7:8
There is increase of 40%, 50% and 75%
New ratio of seats:
5`\times ` 140% : 7`\times `150% : 8`\times ` 175%
7:`\frac { 21 }{ 2 } `:14
2:3:4
Hence the new ratio of seats is 2:3:4
Solution:
Given è Ratio of fares = 4:1
Ratio of number of passengers = 2:17
Ratio of amount collection = 4`\times `2 : 1`\times `17 = 8:17
Hence amount collected from second-class passengers is
`(\frac {17 }{ 25 } )\times ` 2500 = 1700
Required amount collection is Rs. 1700
Solution:
Let us consider the total weight be ‘x’
Acc. to ques. Weight is proportional to square of weight
`x^{ 2 }` = 1296
X = 36
After breakage ratio of weight = 1:2:3
Actual Weight = 6, 12, 18
Total value = `6^{ 2 }` + `12^{ 2 }` + `18^{ 2 }`
= 36+144+324 = 504
Loss after breakage = 1296 – 504 = 792
For two ratio to be equal there is requirement of four variables.
If `\frac { a }{ b } = \frac {c }{ d } ` then a, b, c, d are said to be in proportion
This is expressed as ‘a’ is to ‘b’ is to ‘c’ is to ‘d’
And written as a:b :: c:d
( product of means = product of extremes)
a:b = b:c
Middle number b is called mean proportion and a and c are called extreme numbers.
`B^{ 2 }` = A`\times `C
`B^{ 2 }` = A`\times `C
`12^{ 2 }` = 6 `\times ` C
C = 24
The ratio in the mathematical term used to compare two similar quantities expressed in the same units.
The ratio of two numbers ‘x’ and ‘y’ is denoted as x:y
Note: Fractions and ratio are same but the only difference is that ratio is unit less quantity but fraction is not.
Basics Properties of Ratio
- A:B = mA : mB where m is constant
- a:b:c = A:B:C is equivalent to `\frac { a }{ A } = \frac { b }{ B } = \frac { c }{ C } `
- This property has to be used in the ratio of three things
- The inverse ratios of two equal ratios are equal. This property is called invertendo.
- If `\frac { a }{ b } = \frac { c }{ d } then \frac { b }{ a } = \frac { d }{ c } `
- The ratio of antecedents and consequents of two equal ratio are equal. This property is called Alternendo.
- If `\frac { a }{ b } = \frac { c }{ d } then \frac { a }{ c } = \frac { b }{ d } `
Componendo:
If `\frac { a }{ b } = \frac { c }{ d } then \frac { (a+b) }{ b } = \frac { (c+d) }{ d }`Dividendo:
If `\frac { a }{ b } = \frac { c }{ d } then \frac { (a-b) }{ b } = \frac { (c-d) }{ d }`Componendo-Dividendo:
If `\frac { a }{ b } = \frac { c }{ d } then \frac { (a+b) }{ (a-b) } = \frac { (c+d) }{ (c-d) }`RATIO TRICKS
Trick 1: Splitting number in the given ratio
1. Divide 900 in the ratio 4:5We cannot use x for solving

Total parts = 4 + 5 = 9
First number = `(\frac { 4 }{ 9 })`900 = 400
Second number = `(\frac { 5 }{ 9 })`900 = 500
2. Divide 1500 in the ratio 5:7:3

Total parts = 5 + 7 + 3 = 15
First number = `(\frac { 5 }{ 15 })`1500 = 500
Second number = `(\frac { 7 }{ 15 })`1500 = 700
Third number = `(\frac { 3 }{ 15 })`1500 = 300
TRICK 2: Directly Proportional
When two parameters are directly proportional if one parameter increases the other one also increases and if one decreases another one also decreases.
If parameters A and B are directly proportional then they will satisfy the following equation
`\frac { A1 }{ A2 } = \frac { B1 }{ B2 } `If parameters A and B are directly proportional then they will satisfy the following equation
Example:
Price of diamond is directly proportional to its weight. If 3 grams of diamond costs Rs 45000. Then what will be the price of 8 grams of the diamond.Solution:
As two parameters which are weight and cost are directly proportional.
Hence, `\frac { W1 }{ W2 } = \frac { C1 }{ C2 } `
`\frac { 3 }{ 8 } = \frac { 45000 }{ C2 } `3/8 = 45000/C2
C2 = 120000
TRICK 3: Inverse Proportional
When two parameters are inversely proportional if one parameter increases then other one decreases and if the one decreases then the other increases.If parameter A and B are inversely proportional then they will satisfy the following equation
`\frac { A1 }{ A2 } = \frac { B1 }{ B2 } `
TRICK 4:
If the ratio’s A:B and B:C are given then we can find A:C without solving any equation\begin{matrix} A: & B: & C \\ x & y & y \\ m & m & n \end{matrix}
A: B: C → x` \times ` m : y ` \times ` m : y ` \times ` n
Examples:
If the ratio of A:B is 4:5 and the ratio of B:C is 3:4. Then find the ratio A:C\begin{matrix} A: & B: & C \\ 4 & 5 & 5 \\ 3 & 3 & 4 \end{matrix}
A:B:C → 4` \times `3 : 5` \times `3 : 5` \times `4
A:B:C → 12:15:20
If the ratio of a salary of A and B is 2:3, B and C is 4:5, C and D is 6:7. If the salary of A is 48000 then Find the salary of D.
\begin{matrix} A: & B: & C: & D \\ 2 & 3 & 3 & 3 \\ 4 & 4 & 5 & 5 \\ 6 & 6 & 6 & 7\end{matrix}
A:B:C:D à 48: 72: 90: 105
A:D = 48:105
`\frac { A }{ D }` = `\frac { 48 }{ 105 }`
`\frac { 48000 }{ D }` = `\frac { 48 }{ 105 }`
D’s salary = 105000
TRICK 5: USAGE OF COMMON FACTOR ‘X’
Common factor “X” should be used only when there is no direct relation with ratio either directly or inverselyExample:
Two numbers are in the ratio 3:5. Sum of their squares is 30600. Find the numbers.Ratio ⟶ 3: 5
Assume the actual number 3x and 5x
According to the question,
`(3x)^{ 2 }` + `(5x)^{ 2 }` = 30600
`34x^{ 2 }` = 30600
`x^{ 2 }` = 900
x = 30
Substitute the value of x in 3x and 5x to find numbers
Hence the numbers are 90 and 150
TRICK 6: COIN PROBLEMS
Quantity Ratio ⟶ Q1 : Q2 : Q3Value Ratio⟶ V1 : V2 : V3
(Q1` \times `V1) + (Q2` \times `V2) + (Q3` \times `V3) = T
Common factor X = Total Amount/ T
Quantity of each coin = Q1X , Q2X, Q3X
Example:
1. A bag contains 5 paisa, 10 paisa and 20 paisa coins in the ratio 2:4:5. Total amount in the bag is Rs. 4.50 How many coins are there of 20 paisa?Solutions:
T = (5` \times `2) + (10` \times `4) + (20` \times `5)
T = 150 paisa = Rs. 1.50
X = `\frac { 4.50 }{ 1.50 }`4.50/ 1.50 = 3
Hence the quantity of 20 paisa coins = 5 ` \times ` 3 = 15 coins
2. The bag contains 440 coins of 1 rupee, 50 paisa and 25 paisa and their value are in the ratio 6:7:9. Find the number of 50 paisa coins.
Solution:
Value Ratio ⟶ 6:7:9
Coins Ratio ⟶ 6` \times `1: 7` \times `2: 9` \times `4
3:7:18
Number of 50 paisa coins = `(\frac { 7 }{ 28 }) \times ` 440 = 110
Examples of Ratio
We have explained some of the tricks of ratio in the first part of Ratio. Now we will discuss some more examples of the previous tricks of Ratio.Example 1.
If A:B is 2:5 and B:C is 7:3 then find A:B:C
\begin{matrix} A: & B: & C \\ 2 & 5 & 5 \\ 7 & 7 & 3 \end{matrix}
A:B:C = 7`\times `2 : 7`\times `5 : 5`\times `3
A:B:C = 14:21:15
\begin{matrix} A: & B: & C \\ 2 & 5 & 5 \\ 7 & 7 & 3 \end{matrix}
A:B:C = 7`\times `2 : 7`\times `5 : 5`\times `3
A:B:C = 14:21:15
Example 2.
A wooden stick is broken into two parts one bigger and one smaller part. The ratio of the bigger part and the smaller part is proportional to the ratio of lengths of the full stick and bigger part. Find the ratio.Solution:
Let us consider the length of bigger part be 1 m and the smaller part be ‘x’ m.
So length of full stick = (1+x) m
Acc. to the ques.
`\frac { 1 }{ x } ` = `\frac { 1 + x }{ x } `
`x^{ 2 }` + x = 1
x = `\frac { (-1+\sqrt { 5 } ) }{ 2 }` (As the length will be positive)
Example 3.
Three persons A,B,C whose salary together amount to Rs. 96000. But the expenses of all three person is 75% , 85% and 80% of their salaries. If their savings is in the ratio of 8: 9:20. Find the salary of BSolution:
Let us suppose the salary of A be Rs. x, B be Rs. y and C be Rs. Z
Then the savings of them is
`\frac { (x\times 25)}{100}`, `\frac { (y\times 15)}{100}`, `\frac { (Z\times 20)}{100}`
`\frac { x }{ 4 }` , `\frac { 3y }{ 20 }`, `\frac { z }{ 5 }` = 8:9:20
x:y:z = 32:60:100 = 8:15:25
B’s salary will be `(\frac { 15 }{ 48 })\times ` 96000 = Rs. 30000
Example 4.
Seats in medical, commerce and art department in the college is in the ratio of 5:7:8. But due to the popularity of the college, management decided to increase the number of seats by 40%, 50% and 75% respectively. Find the new ratio of a number of seats available.Solution:
Initial ratio of seats = 5:7:8
There is increase of 40%, 50% and 75%
New ratio of seats:
5`\times ` 140% : 7`\times `150% : 8`\times ` 175%
7:`\frac { 21 }{ 2 } `:14
2:3:4
Hence the new ratio of seats is 2:3:4
Example 5.
The ratio of the first and second class fares between two railway stations in 4:1 and that of the number of passengers is 2:17. If on a day Rs. 2500 is collected as a fare. Find the amount collected from the second class passengers.Solution:
Given è Ratio of fares = 4:1
Ratio of number of passengers = 2:17
Ratio of amount collection = 4`\times `2 : 1`\times `17 = 8:17
Hence amount collected from second-class passengers is
`(\frac {17 }{ 25 } )\times ` 2500 = 1700
Required amount collection is Rs. 1700
Example 6.
Price of the diamond is directly proportional to the square of its weight. If the diamond is dropped and broken into three pieces of the ratio 1:2:3.If the price of original diamond is Rs. 1296 Find the loss after breakage of diamondSolution:
Let us consider the total weight be ‘x’
Acc. to ques. Weight is proportional to square of weight
`x^{ 2 }` = 1296
X = 36
After breakage ratio of weight = 1:2:3
Actual Weight = 6, 12, 18
Total value = `6^{ 2 }` + `12^{ 2 }` + `18^{ 2 }`
= 36+144+324 = 504
Loss after breakage = 1296 – 504 = 792
Some Examples:
- A person covers a certain distance by train, bus and car in ratio 4:3:2. The ratio of the fare is 1:2:4 per km. The total expenditure as a fare is Rs.720. Find the total expenditure as fare on car
- A sum of money is distributed between A, B, C and D in the ratio 1:3:6:7. If the share of D is Rs.3744 more than A. Find the total amount of money of B&C together?
- A bag contains 1 rupee, 50 paisa and 25 paisa coins in the ratio 2:3:5. Their value is Rs.288. Find the value of 50 paisa coins.
- The salary of A,B, C are in the ratio of 2:3:5. If the increment of 10%, 15& and 20% are allowed respectively on new year. Find the ratio of new incremented salaries of A,B,C.
- In a business, A and C invested in the ratio 2:1 whereas the ratio between amount invested by A and B was 3:2. If Rs.1,57,300 was their profit. Find the amount invested by B.
Proportion
The numbers or quantities are said to be in proportion when the two ratio between them are equal.For two ratio to be equal there is requirement of four variables.
If `\frac { a }{ b } = \frac {c }{ d } ` then a, b, c, d are said to be in proportion
This is expressed as ‘a’ is to ‘b’ is to ‘c’ is to ‘d’
And written as a:b :: c:d
( product of means = product of extremes)
Mean or Third Proportion
When there are only three variables or quantities like a, b, c. Then the middle number is to be repeateda:b = b:c
Middle number b is called mean proportion and a and c are called extreme numbers.
`B^{ 2 }` = A`\times `C
Example 1.
Find the third proportion of 6 and 12
Given it is third proportion where a = 6 and b = 12`B^{ 2 }` = A`\times `C
`12^{ 2 }` = 6 `\times ` C
C = 24
Some Examples:
- The fourth proportion to 75, 192 and 200 is equal to fourth proportion to 90, 384 and Q. Find the value of Q.
- What is the value of ‘x’ if it is 45% of the fourth proportion of 5, 8 and 25
- By how much the fourth proportion to 26, 143 and 68 will be greater than third proportion to 49 and 63?
- Find the mean proportion between (3+√2) and (12-√32)
- What is the least number must be subtracted from the numbers 14, 17, 34 and 42 so that the numbers may be in proportion?

